An Introduction to Constant Proportion Portfolio Insurance (CPPI) products
Constant Proprtion Portfolio Insurance or CPPI products are capital guarantee product based on a dynamic asset allocation strategy. The strategy actively allocates between two asset classes - a riskless asset and a risky asset which could be from equity, hedge funds, funds, equity or commodity indices etc. In rising markets the strategy allocates more towards the risky asset while in falling market, the strategy allocates more towards the safe asset. CPPIs are also one of the most popular derivative products because of their capital protection feature. Although CPPIs were first developed for the retail investors, they are now popular even with pension funds and insurance companies as the structure still ensures minimum future cash flow required by these industries.
Understanding the Payoff
Suppose we have a CPPI product expiring in 5 years time and the buyer has invested £100 worth CPPI. Also lets assume that £90 worth zero coupon bonds today will yield £100 in 5 years time. CPPI products allocate to risky asset based on the pre-specified Multiplier (or leverage). So lets say that the Multiplier is 4, then the strategy allocates 4*(£100-£90)=£40 to the risky asset initially and the rest £100-£40=£60 to riskless bonds. If now lets say that the market rallies so that the new portfolio (risky asset + riskless asset) value is £105, the strategy will now allocate 4*(£105-£90)=£60 to the risky asset whereas now only £40 to bonds. On the other hand if the market falls so that the new portfolio value is £95, the strategy will now allocate 4*(£95-£90)=£20 to risky asset whereas now £80 to bonds. By allocating more and more progressively to the riskless bond when the market falls, the strategy ensures capital protection (on most ocassions - see discussion on GAP risk below).
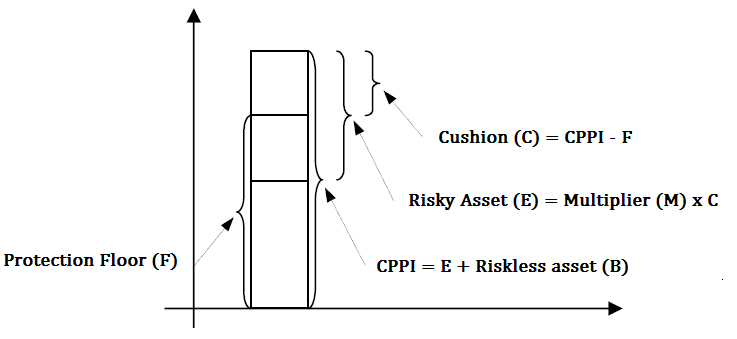
In the example above, £90 is the amount the strategy requires to guarantee the principal £100 back at maturity and is termed as the Protection or Bond Floor. The investment in Protection or Bond Floor is £40. The excess of portfolio over the protection floor is referred to as the Cushion amount (=£10 in the example above). If at certain stage the portfolio value declines to the protection floor level, the strategy allocates completely to the riskless asset and will stop investing in the risky asset for rest of the duration of the product. This situation is sometimes called "cash lock".
Comparison to bond + a call investment
CPPIs are sometimes compared to a bond + a call option capital protected strategy. In the example above, lets say that £90 were invested in a riskless asset so that they yield £100 at maturity and the rest £10 giving you participation in the risky market through a call option. While the exact difference in the payoff of a CPPI and this bond+call option strategy will depend on the path taken by the risky asset along with its value at maturity, we can make a few comments on how their payoffs compare in different market environments. In rallying market, its straightforward to see that the CPPI product will outperform the call + bond investment strategy because the CPPIs would progressively increase the allocation to the risky asset giving you more and more exposure. In falling markets both the strategies would yield back the principal at maturity. On occasions where the risky asset falls below the Protection floor and then rebounds above its initial level, the payoff of a call + bond would yield a higher payoff as the CPPI strategy would have allocated everything to the riskless asset cutting off the exposure in the risky asset for rest of the life of the product.
Negative gamma product
CPPIs are negative gamma products for the buyer. To replicate the product, one would be buying the risky asset in the rising markets while selling in falling markets i.e. buying high and selling low.
GAP Risk
If the prices of the risky asset change gradually then the seller has sufficient time to deallocate from the risky asset and allocate to the riskless asset in case the markets start collapsing. However a critical situation can occur if there is a dramatic fall in the value of the risky asset between two rebalancing dates. In worst possible scenario, the CPPI portfolio can fall below the Protection floor before the manager could rebalance. In such a situation the CPPI portfolio would loose its capital protection. We notice that higher the value of Multiplier (M) higher would be the GAP risk. (1/M) is often referred to as the GAP size. It refers to the maximum loss that could be sustained between two rebalancing date before the portfolio breaks the protection floor. In the example above M=4, thus risky can fall upto 1/4 =25% between two rebalancing dates before the CPPI portfolio falls to the Protection floor risking the capital protection.
To minimise the GAP risks it is essential to select the risky asset carefully (use liquid and less volatile assets). The multiplier should be small to keep the GAP risk limited yet large enough to provide decent leverage. Also by increasing the frequency of rebalancing the GAP risk can be kept under control.
Interest rate risk
So far we have been guilty of using the term "riskless" for the non-risky asset in the CPPI structures. However these non risky assets bear interest rate risks that influence the Protection floor (the amount required to bear the principal at maturity) and hence the cushion and the amount invested in the risky asset.
Pricing and hedging considerations
To account for the GAP risk, the pricing methodology should incorporate abrupt movements or "jumps" in the price movements. Often the seller guarantees the capital protections so that he bears the GAP risk of the product. If the risky asset is a liquid one then he sometime hedges the GAP risk by buying and rolling OTM put options on either the underlying asset or a correlated index. If however the risky asset is not a liquid one, buying and then hedging put options would not be easy and hence it would be prudent for him rather to keep the Multiplier as small as possible than investing in put options.









